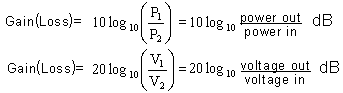|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Syllabus Sections:- Decibels 3o.1 Understand the equations for decibel power and voltage ratios.
The equations are :-
When assessing the sound level strength of an audio signal this is how loud it seems to be when heard by the human ear. If someone thinks that a sound level has doubled when power from a audio amplifier is raised from 5 to 10 watts that same person should also consider that a signal would also be twice as loud if the power increased from 200 to 400 watts. The human ear has what is called a "logarithmic" response to changes in audio levels. It is this basis that is used for the "relative" power level given the name of "the decibel" (dB). One decibel is 1/10th of a bel the unit of sound coined by Alexandra Graham Bell. The number of decibels in relation to the ratio of power levels is given by :-
When using the V1 / V2 voltage equation the the impedance must be the same for both voltages, thus a power amplifier cannot be properly assessed if only taken on input and output voltages, unless those voltage have the same value of impedance in the circuit. These equations look very complex but in the scientific calculator sections it is shown how the equations can be used when P1 = PowerOut P2 = PowerIn similarly V1 = VoltsOut and V2 = VoltsIn
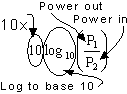
We are using log to the base 10 as shown by "log10" the 10 to the right of the word log indicate base 10. For clarity we will now omit that 10 and only write "log". When a question is set and Powerin is not shown then assume that it is 1 Watt. Example: What is a measured power of 400W expressed in dBW ? dBW = 10 log(power in watts) dBW = 10 log(400) = 26dBW Click here to see the calculator key strokes Example: What is a measured power of 12dBW expressed in Watts ? Power(Watts) = antilog dBW/10 Power(Watts) = antilog 12/10 16 Watts Click here to see the calculator key strokes
There is another equation that is given in the equation listing.
This is used to determine the gain of a yagi over a dipole using the power from each.
Recall (or
determine) the power gain or loss of various dB ratios based on ± 3,
6, 9, 12, 15 and 10, 20, 30dB. (This includes examples such as 25W
The sort of question you might be asked is:- Your transmitter has a power output of 20dBW and the feeder has a loss of 3dB, the antenna has a forward gain of 9dB. What is your ERP in watts (effective radiated power) ? Solution 20dBW - 3 + 9 = 26dBW Now we know that Power(Watts) = antilog dBW/10 so Power(Watts) = antilog 26/10 = 398W rounded up = 400W Click here to see the calculator key strokes
|
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||